Topological insulators get down to business

Go to any condensed-matter physics meeting, and topological insulators are prominent on the agenda, and talks on the topic attract large audiences. For good reason. Topological insulators promise nothing less than a revolution in electronics. Even though as their name suggests topological insulators are electrically insulating, they are conducting on their surface. And unlike regular conductors, these surface currents flow without the electrons being thrown off the track by most (albeit not all) scattering effects from impurities. This is one of their key features that ultimately may lead to smaller and faster electronic devices.

Even though first experimental breakthroughs have been achieved since 2006 in two-dimensional (thin films) of HgTe with similar properties, the tell-tale surface currents haven’t been observed in three-dimensional topological insulators such as the widely studied Bi₂Se₃ and Bi₂Te₃. So far, samples have not reached a sufficient purity and researchers had to make do with indirect characterisation experiments rather than direct measurements of electrical transport. This has now changed. In a study published in today’s issue of Science, Robert Cava, Nai Phuan Ong and colleagues from Princeton University report on the first experiments demonstrating electron conduction on the surface of Bi₂Te₃.

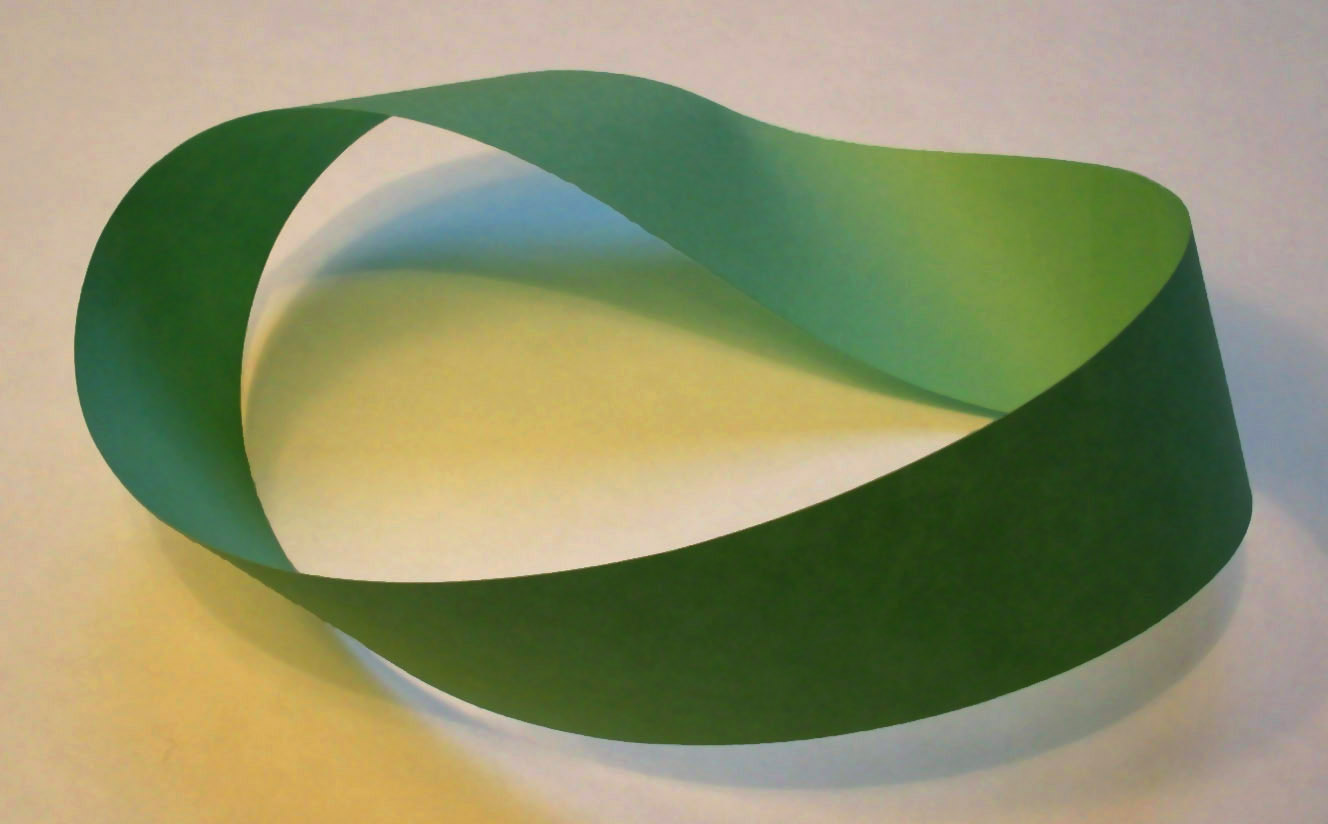
A Möbius strip retains it topological properties even if deformed. It doesn't become a conventional rubber band and vice versa. The electronic properties of a topological insulator are similarly protected against perturbations. Photograph by David Benbennick via Wikimedia Commons.

From a mathematical point of view, crystals are perfectly periodic objects that stretch out infinitely. Real materials on the other hand always have a surface where atomic bonds are left dangling without a corresponding partner at the other end. Therefore, surface electronic states are generally different to those within the crystal.

In many electronic devices these surface states are a real nuisance. In solar cells, they often reduce the light conversion efficiency. In a topological insulator, surface states are the key feature.

Different to most compounds, the surface states in a topological insulator are protected against external disturbances. This is through their special topology. This can be illustrated by the comparison of two objects with different topology, such as a regular rubber band and a Möbius strip. You cannot transform a rubber band into a Möbius strip without cutting it, and vice versa. The same is true for the surface states of a topological insulator, they are stabilized by their topology and unlike conventional surface states do not alter their character even when subject to perturbations.

This protection arises from time-reversal symmetry of the topological states. That is, the system behaves the same way, regardless how time flows. This is different to the macroscopic world, where time-reversal generally doesn’t happen. If you play a video tape backwards, motions will look very different. In a topological insulator, it would look the same no matter how you play the tape.

As a consequence of the topological protection, scattering from impurities that would destroy the topological states does not occur (as long as these impurities are non-magnetic, because magnetic fields mess with the time-reversal symmetry of the topological state). This is down to the effects of quantum physics where in case of time-reversal symmetry the clockwise or counterclockwise paths that an electron can take around an impurity cancel each other out, so that the overall probability of a particle being scattered is zero.

So far, surface currents in three-dimensional topological insulators have not been demonstrated. Purity of the samples has been a major issue, and crystals were not insulating at all. To obtain insulating samples, the researchers now grew crystals of Bi₂Te₃ with slightly varying composition of elements throughout the sample to be sure to hit the right balance. They then cut the sample in thin slices and looked for pieces that while not perfectly insulating at least showed a sufficiently suppressed electrical conductance in their interior to look for surface effects. Indeed, in some of their samples  the researchers were able to confirm the presence of an electrically conducting surface layer where the electrons move about 12 times faster than those in the interior.

However, these experiments, exciting as they are, are merely the beginning. As a next step, sample quality needs to improved even further so that key properties specific to topological insulators can be verified, such as the quantum spin Hall effect. A number of exotic effects predicted by theoretical physicists also await experimental confirmation. One example better dealt with in a later, separate blog post are Majorana fermions, which emerge at the interface of a topological insulator and a superconductor. The party has only started.

Reference:
Qu, D., Hor, Y., Xiong, J., Cava, R., & Ong, N. (2010). Quantum Oscillations and Hall Anomaly of Surface States in the Topological Insulator Bi2Te3 Science, 329 (5993), 821-824 DOI: 10.1126/science.1189792

Further reading: Free access features appeared in Physics, arXiv, Nature, and Uncertain Principles.