Fibre optic cables transmit information so fast because they can make use of the unique properties of light and transmit many data channels at the same time. The digital 1s and 0s the light beams carry are imprinted onto the beams by semiconductors that in quick succession turn the light beam on and off. Unfortunately, that also puts a limit on the possible data rate, as materials switch slower than light. There are all-optical switches operating at the speed of light using special crystals, but what is needed are solutions that can be fabricated on a chip.
This is made possible now. Georgios Ctistis, Willem Vos, Jean-Michel Gérard and colleagues from the University of Twente and the FOM-Institute Amolf in the Netherlands, and the Institute for Nanoscience and Cryogenics in Grenoble in France have demonstrated that using a material to switch light is not a drawback anymore. They are able to switch a light beam within a semiconductor device at speeds of 0.3 picoseconds, where a picosecond is a millionth of a millionth second. That’s so fast that it approaches the limit set by the speed of light.
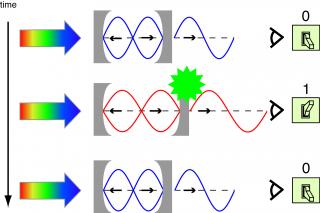
The principle of the ultimate optical switch. Top: a microcavity blocks the transmission of the red signal beam. Middle: in the presence of a control beam the cavity changes its properties and lets the beam pass. Bottom: as the control beam is off again, the switch also turns off. Figure provided by the authors.
In a conventional optical switch, a light beam (or an electrical voltage), is used to excite electrons in a semiconductor. These electrons then change the material’s optical properties in a way that switches the signal beam on or off. But this is a comparatively slow process. The idea here is to separate the optical effects from materials properties, which would only slow the device down. “The key advance is that both the switch-on and -off times of the semiconductor microcavity is completely determined by the properties of light itself,” says Vos.
The way this works is to use a microcavity, where light is confined between two mirrors. The mirrors as well as the microcavity are made of precisely controlled layers of the semiconductors GaAs and AlAs, because these work particularly well for the wavelengths used in telecommunications.
The switching process involves two laser beams. It is important that these two lasers, the signal beam as well as the control beam that triggers the switching, have energies that are below the bandgap energy of the semiconductors. That way, the photons in the laser beam can’t excite electrons in the semiconductor, which as mentioned would slow down the switching. In fact, the energy of the signal beam is even less than half of the bandgap energy, so that there is not even the chance of two photons combining together to excite an electron.
Switching occurs only for that brief moment where the signal and the control beam come together. In that moment, the semiconductor microcavity, which normally would block the signal beam, lets it pass. This is due to an nonlinear optical effect, the so-called electronic Kerr effect. Again, electrons are not really relevant here, as the combined energy of signal and control beam isn’t enough to excite electrons above the bandgap. Rather, the increased light intensity, through the Kerr effect, modifies the refractive index of the semiconductors. This change in materials parameters changes the resonance frequency of the microcavity, so that light that otherwise would be trapped can escape.
Being a non-linear optical effect means that the laser intensities remain quite large says Vos. Therefore, he says, the next step now is to “switch to tiny micron-sized cavities (e.g. micropillars, photonic crystal) with weak pulses from on-chip lasers.” Smaller cavities require less switching power.
Either way, switching speeds on the order of a picosecond correspond to signal frequencies in the THz regime, which is what next generation optoelectronic switches need to achieve. However, at this stage the researchers did not yet show successive switching at such frequencies. That’s one of their next tasks, comments Vos. “We are working hard to demonstrate repetitive switching where consecutive switch events occur every ps or so.” It won’t get much faster than that.
Reference:
Ctistis, G., Yuce, E., Hartsuiker, A., Claudon, J., Bazin, M., Gérard, J., & Vos, W. (2011). Ultimate fast optical switching of a planar microcavity in the telecom wavelength range Applied Physics Letters, 98 (16) DOI: 10.1063/1.3580615


May 1, 2011 at 08:28
I am confused.
In my (non-physicist’s) understanding, the speed of light is a limit to physical travel.
Switching frequency is a fundamentally different concept, isn’t it? Why can’t it get any faster than 0.3 pS? In the present case, it is due to interference – and not any physical movement.
May 1, 2011 at 10:36
Thanks for the question, Bhushit.
Well, the switching requires that the light circulates between the mirrors. Basically, the time the light stays in this microcavity determines how often you can switch. That’s given by the speed of light, and the present experiment approaches this limit. I hope this clarifies this aspect, but please do let me know if you have further questions.
May 4, 2011 at 04:56
Thanks. Now I understand.
The cavity has some size/shape – and for *that* size/shape, there is a limit imposed on switching by the speed of light – and that limit (0.3pS) has reached. This is because the wave has to reflect back for creating complete interference pattern.
In that case, smaller/differently shaped cavities should lead to faster switching still. In this view, this is not a fundamental limit on speed of switching.
I hope I am correct 🙂
May 4, 2011 at 10:25
yes, that’s correct 🙂 but note that there is a minimum size on the cavity – half the wavelength of the laser (otherwise you couldn’t squeeze the light in)
May 9, 2011 at 05:39
… again, the smaller the cavity, the higher the frequency of laser can be deployed 🙂 🙂
‘Blue Ray Disk’ way – too familiar for engineers like me!
BTW,it may not always be possible to jack up frequency in quantum domain.
In my understanding, in quantum domain, frequency relates with energy, which may knock electrons off in undesired fashion.
So, there could be an upper limit to unlimited shrinkage of such cavities on a given material. Am I right, Jeorg?
Moore’s law is perhaps the only law which has more problems with physics than with economics 🙂 🙂
May 9, 2011 at 08:54
well yes of course. As I mentioned in the article, the energies of the light have to stay at less than the band gap of the material, which is in the red/near infrared region of light. Specifically, the design here is aimed at telecommunications frequencies at 1.55 micormeters for transmission through optical fibres, and there won’t be any advantage in going to blue/UV light for example…
May 8, 2011 at 11:10
what an interesting blog, congrats, I wrote a comment on one of the post of your old blog which was also great
May 13, 2011 at 00:51
You got me up to ‘speed’, so to say here. A chemist long ago, I thought physicists were just those guys who wouldn’t get their hands dirty. I lack the means to envisage this stuff, assuming its about trying to speed up ‘communication’. This speed of communication would thus allow faster computation? It also seems to do with topology. I’m genuinely fascinated that I know so little (no pun) or don’t if you follow the brackets!
The ‘bucket chemist’ in me wonders how we can work, non-mathematically, so small. I kind of know from your lucid explanation. No flattery meant, but you triggered gratitude. Quite how we’d fire something into something else, taking account of not triggering … but thanks are due. I can ‘see’ the experiment now and as few papers failed to get me to that.
November 11, 2011 at 15:57
As one of the authors of the described result, I wish to thank Joerg Heber not only for describing our work, but also for his efforts to answer all questions from curious posters (thank you too!) and explaining the stuff much better than us authors could do (we’re probably “too close to the subject” to be able to!) Cheers!
January 10, 2012 at 15:43
Hi,
Thank you for your useful Blog.
Is there any article or paper which is demonstrated about this switching time (0.3 ps) ??
January 10, 2012 at 15:50
Hi Leily
Thanks for the kind comments!
the paper is the one from the “Reference” section above – you can find it here: http://dx.doi.org/10.1063/1.3580615